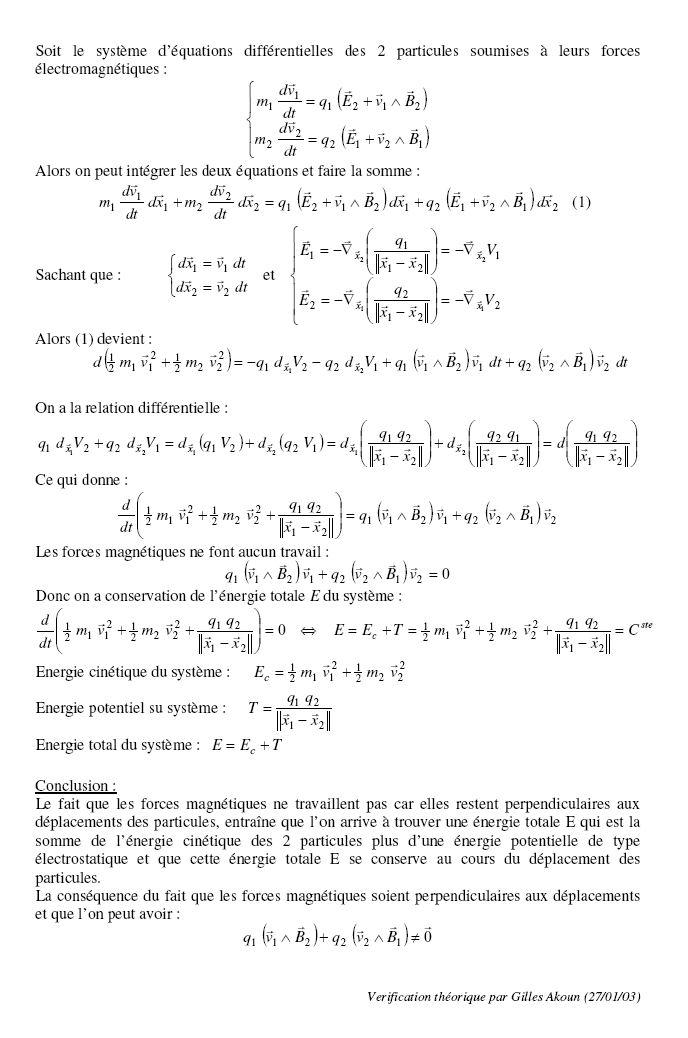
This experiment is fully described in "The Feynman Lectures on Physics" : Electromagnetism vol2, Chap: 26-2 by Addison-Wesley Publishing company or collection InterEdition - ISBN 2-7296-0029-9.

A - The magnetic field created by a charged particle in motion

A charged particle in motion produces a magnetic field that turns around the axis of displacement.

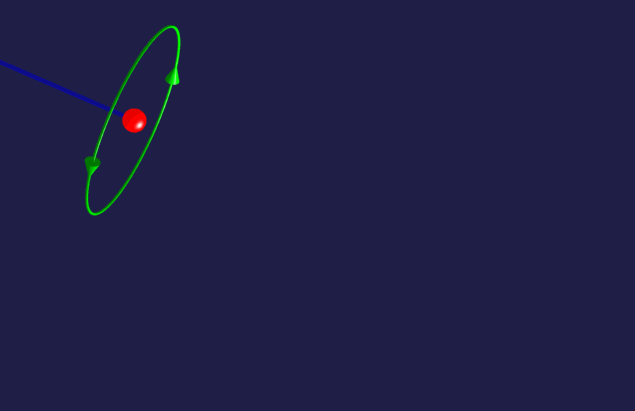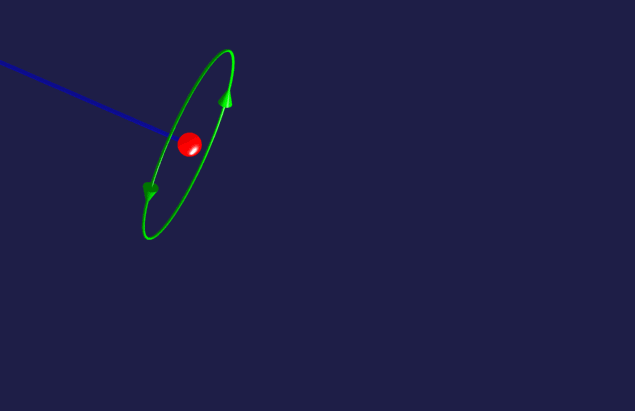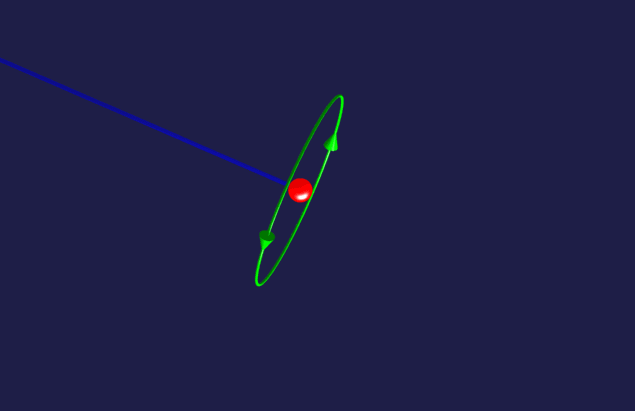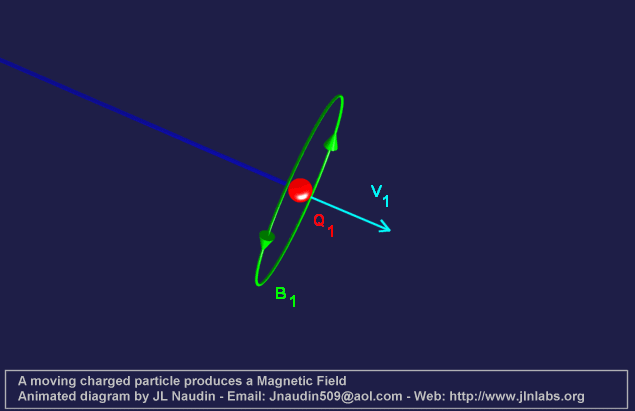
B - The Coulomb and Lorentz force between 2 charged particles in motion

Let us consider the case of two (positively) charged particles with charges Q1 and Q2 respectively, moving with speeds V1 and V2 on mutually intersecting and perpendicular trajectories. The initial position of Q1 is such that Q1 does not collide with Q2, but instead arrives at the intersection point after Q2. 

Look at the animation above carefully.  Note that there is no magnetic field along the trajectory of particle Q1 ( because particle Q2 has arrives at the intersection point before Q1 ).  Let us observe what occurs when particle Q1 arrives near particle Q2 ( at the last stage of the animation ):

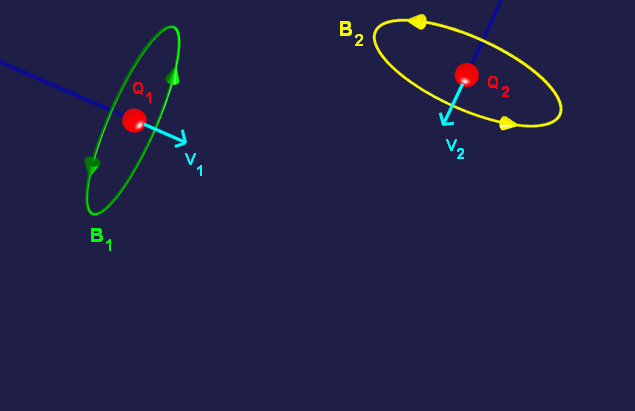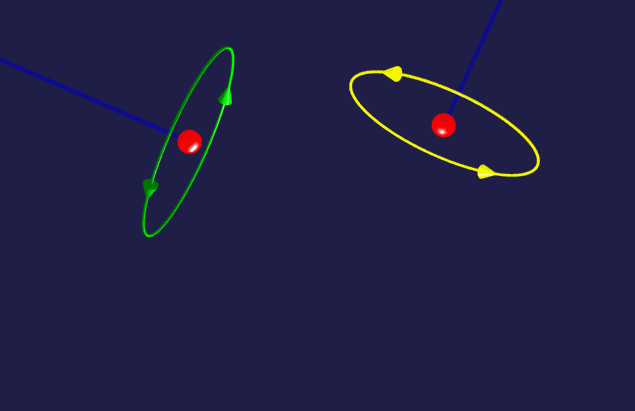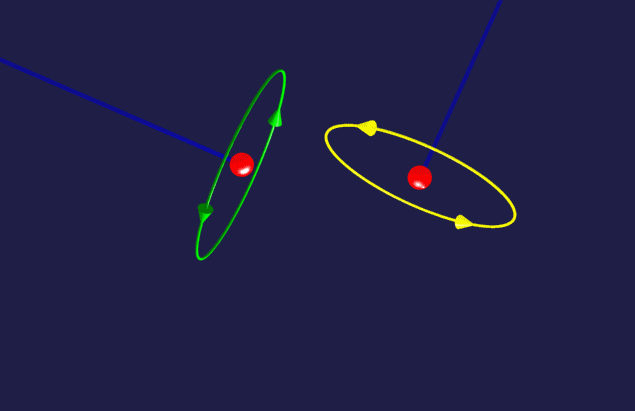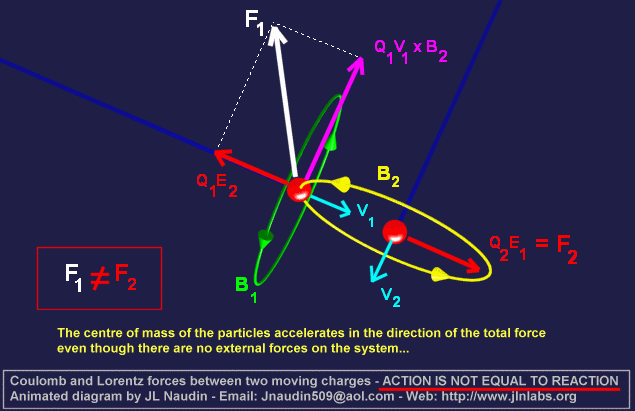
• There is no magnetic field along the axis of Q1, so Q2 is subjected only to the electrostatic Coulomb force produced by the electric field of Q1.

• On the other hand, Q1 is under the influence of the magnetic field produced by Q2. In this case there are 2 forces are involved : the electrostatic force produced by the electric field of Q2 and the magnetic Lorentz force produced by its own displacement through the magnetic field of Q2.

The electrostatic Coulomb forces are equal and opposite, so they validate Newton's 3rd law, however there is a magnetic Lorentz force on Q1 but no magnetic force on Q2.

Here we have a Force of ACTION WITHOUT a Force of REACTION... In this case, Newton's 3rd law is invalidated.

The center of mass of the particles Q1 and Q2 will accelerate in a preferential direction without any external force on the system...

Note : To be in agreement with Newton's 3rd law, it would be necessary to take into account the moments of the magnetic and electric fields.

Reference documents :

• "The Feynman Lectures on Physics" : Electromagnetism vol2, Chap: 26-2 by Addison-Wesley Publishing company or collection InterEdition - ISBN 2-7296-0029-9

• La Physique en MP - PC 1re Année - Volume 3 - Electricité ( électromagnétisme ) par Pierre Alais et Michel Hulin - Librairie Armand Colin.

• Very interesting web site :

  Fundamentals of Ether-based motion  and Inner-ether Energetics by G. P. Ivanov : http://www.tts.lt/~nara/intro.htm